Integration by Partial Fractions
`color{green}"Steps 1 :"` Factor the denominator.
`color{green}"Steps 2 :"` Break up the fraction on the right into a sum of fractions, where each factor of the denominator in Step 1 becomes the denominator of a separate fraction.
`color{green}"Steps 3 :"` Multiply both sides of this equation by the denominator of the left side.
`color{green}"Steps 4 :"` Take the roots of the linear factors and plug them one at a time into x in the equation from Step 3, and solve for the unknowns.
`color{green}"Steps 5 :"` Plug these results into the A and B in the equation from Step 2.
`color{green}"Steps 6 :"` Split up the original integral into the partial fractions from Step 5 and integrate.
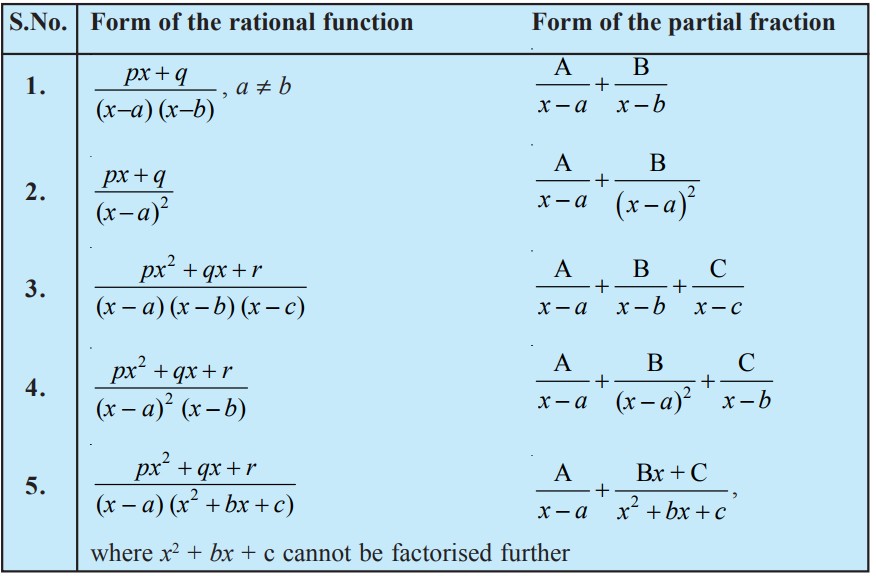
The following Table indicates the types of simpler partial fractions that are to be associated with various kind of rational functions.
`color{green}"Steps 2 :"` Break up the fraction on the right into a sum of fractions, where each factor of the denominator in Step 1 becomes the denominator of a separate fraction.
`color{green}"Steps 3 :"` Multiply both sides of this equation by the denominator of the left side.
`color{green}"Steps 4 :"` Take the roots of the linear factors and plug them one at a time into x in the equation from Step 3, and solve for the unknowns.
`color{green}"Steps 5 :"` Plug these results into the A and B in the equation from Step 2.
`color{green}"Steps 6 :"` Split up the original integral into the partial fractions from Step 5 and integrate.
The following Table indicates the types of simpler partial fractions that are to be associated with various kind of rational functions.












